What do you notice?
What do you wonder?
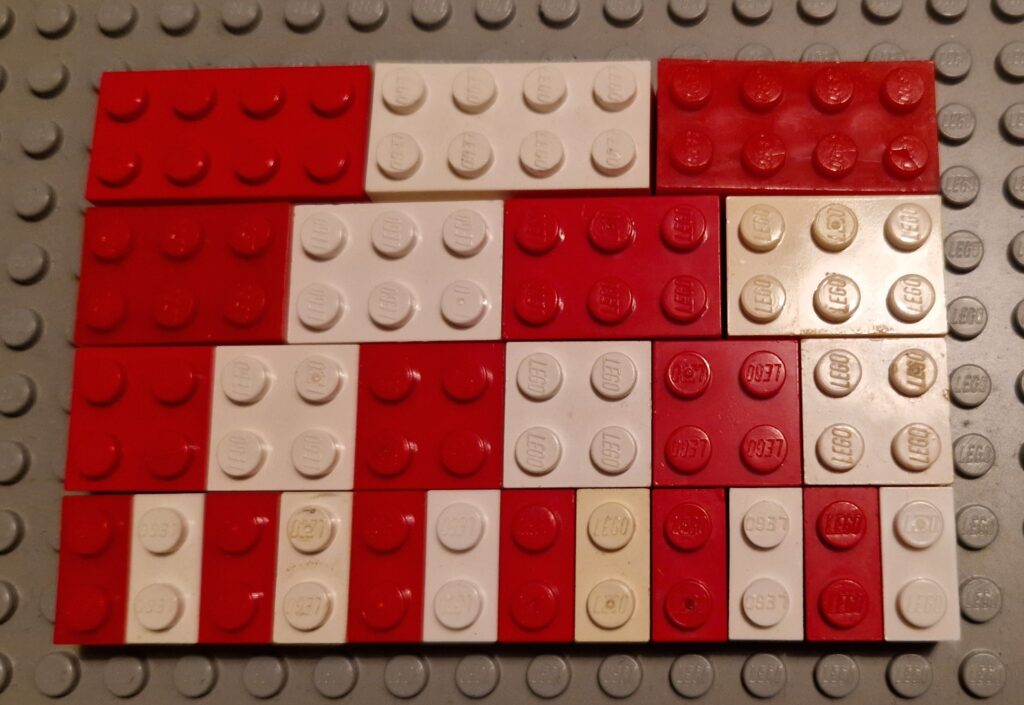
Can you see any fractions in this picture?

Consulting mathematician
What do you notice?
What do you wonder?

Can you see any fractions in this picture?
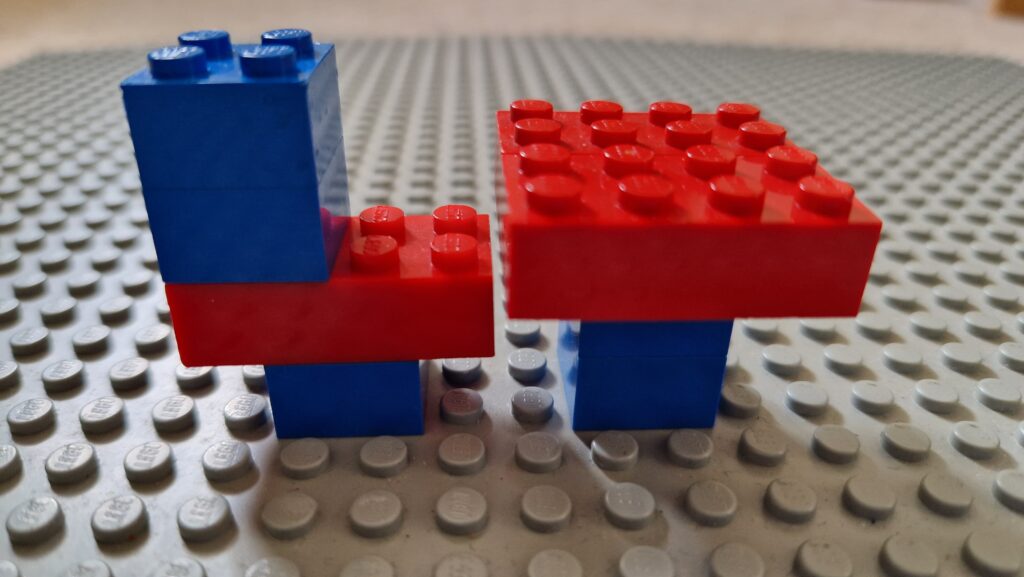
Here is a table and chair made from red and blue bricks. How many red bricks can you see? How many blue bricks?
Explore how many red and blue bricks you would need to make different sets of tables and chairs!
Is there more yellow, more red, or both the same?
Is this part of a pattern? How might the pattern continue?
How many bricks will there be in the next pattern?
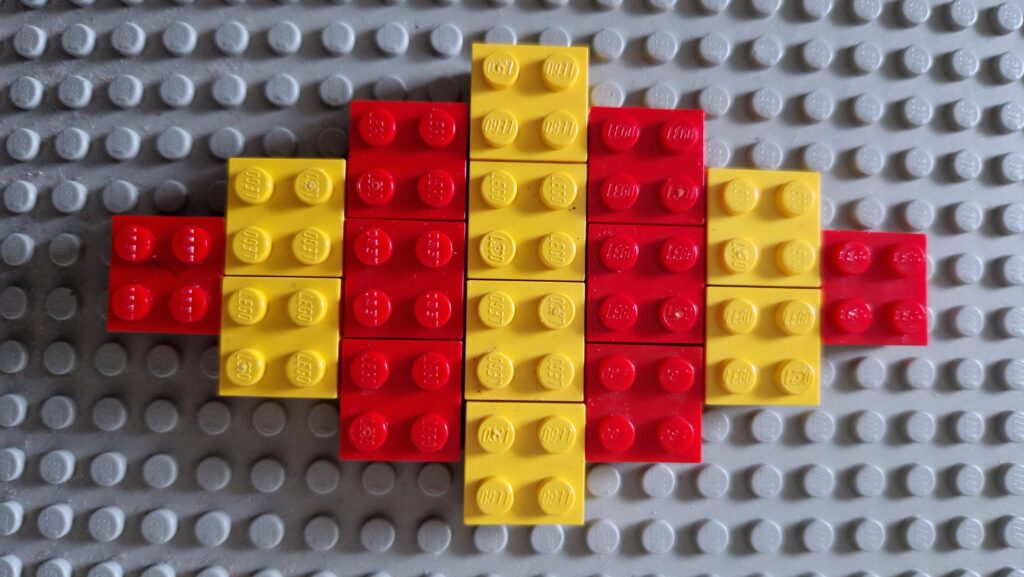
What other mathematical questions could you explore?
The pattern below has 7 rows of red and yellow bricks.
Can you work out the sequence?
Can you describe what the eighth row would look like?
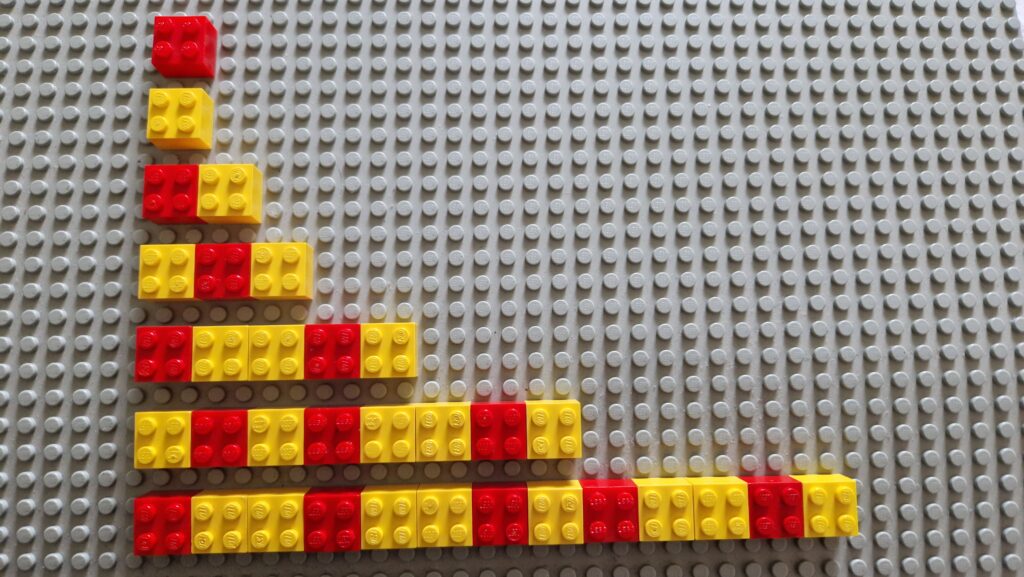
Is there a quick way to work out how many reds and yellows you would need for future rows?
Here is a ‘circle’ made out of Lego. It’s not very circular!
What questions might a mathematician ask?

What is the ‘radius’ of this circle? What is the area?
How could you make a better circle out of Lego?
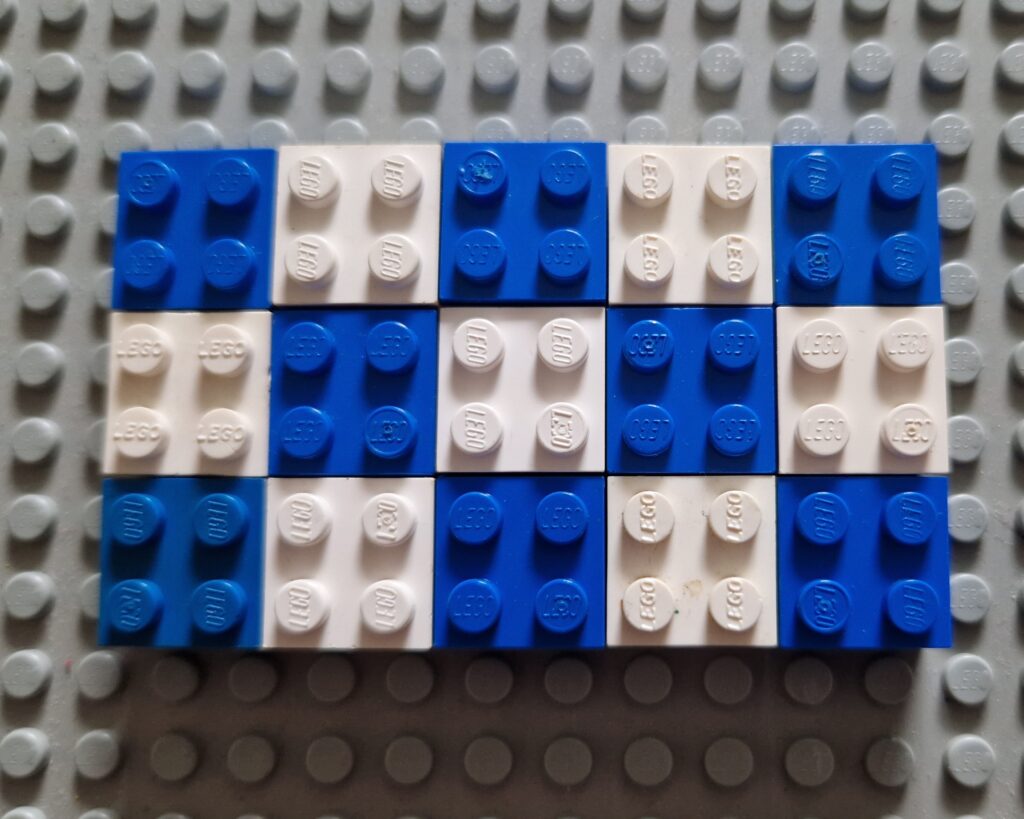
Are there more blue bricks or white bricks? Can you work it out without counting?
This is a 3 by 5 pattern. Can you work out how many blue and how many white bricks you would need for other sizes of pattern?
Have you ever seen other patterns like this?
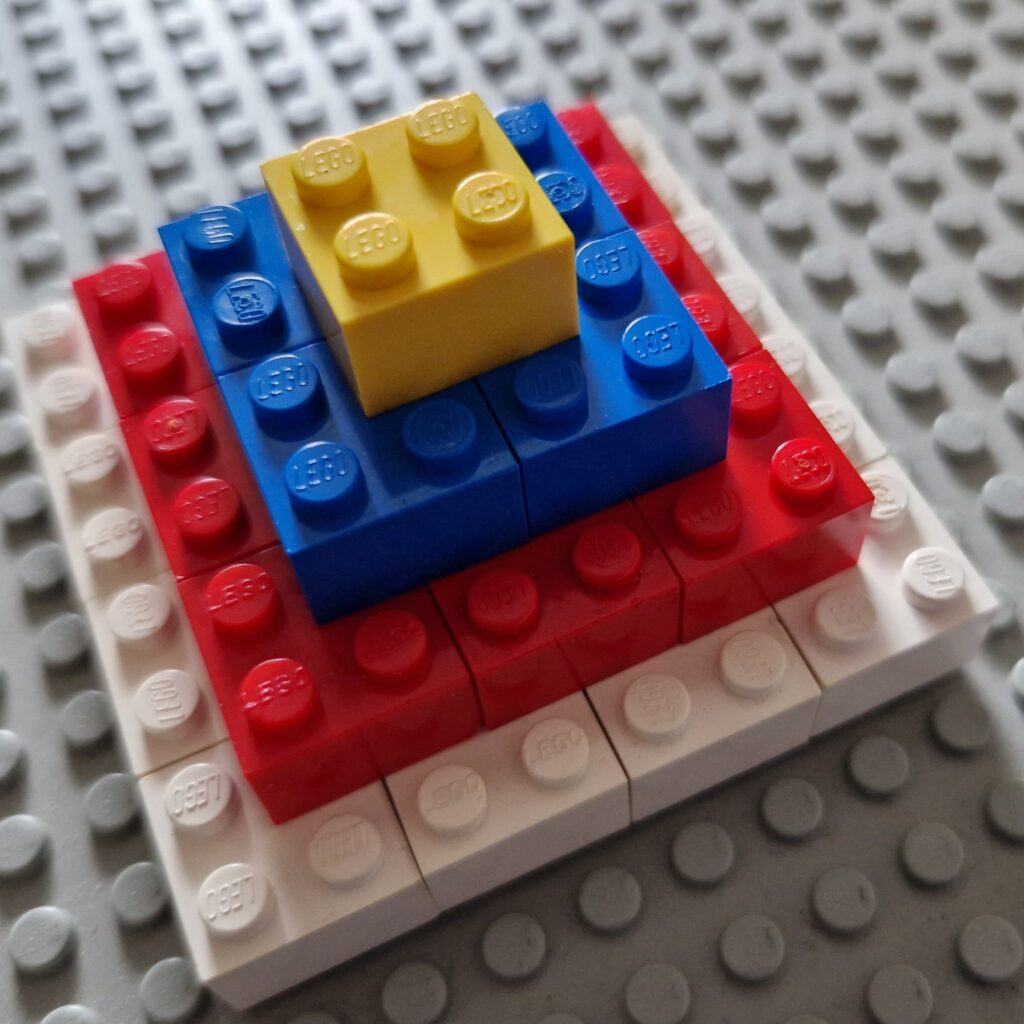
How many bricks?
Can you estimate it? Can you work it out exactly?
What assumptions did you make?
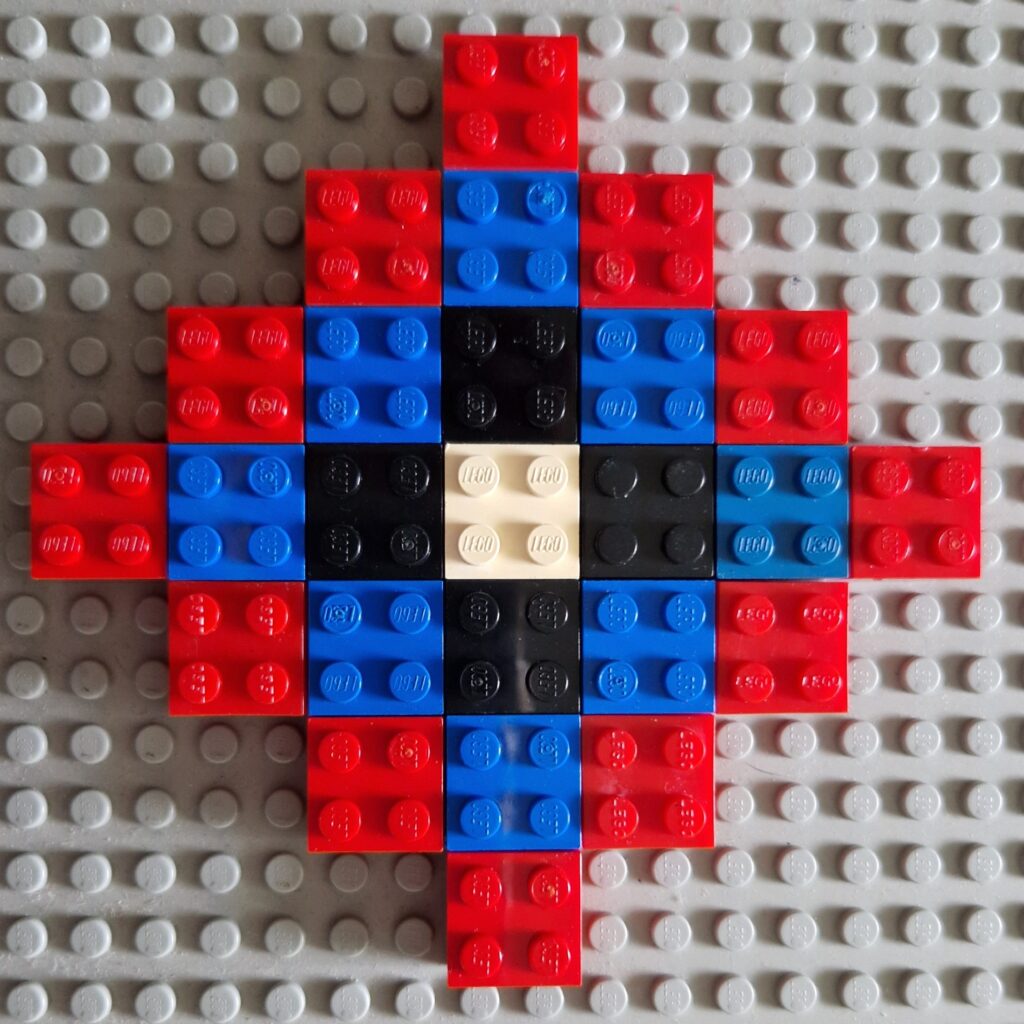
What do you notice?
What do you wonder?
If this shape was part of a sequence, what position in the sequence might it be? What other shapes would appear in the sequence?
Is there a quick way to work out how many bricks you would need for different shapes in the sequence?
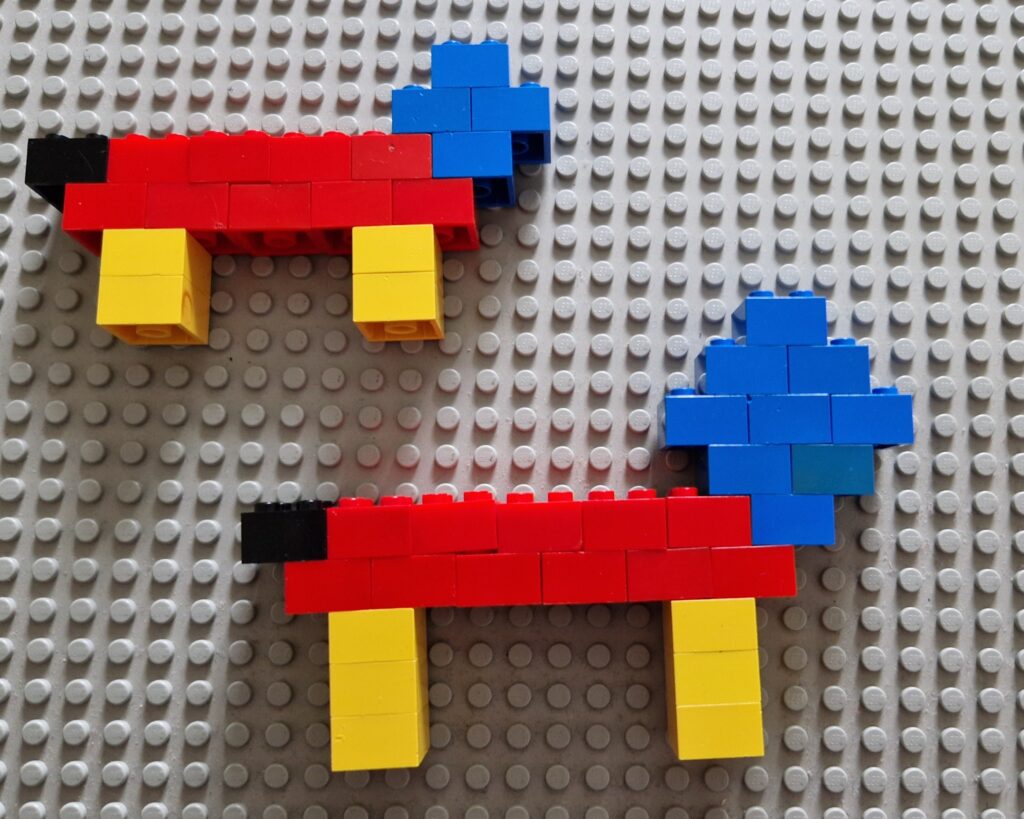
Here are two animals made out of lego. (What animals could they be?)
Can you describe or build the next animal in the sequence?
How many red bricks would you need?
How many blue bricks?
How many yellow bricks?
How many black bricks?
Can you predict how many bricks of each colour you would need if the sequences continued?
Can you make some Lego animal sequences of your own?
Here is the fourth pattern in a sequence made of Lego.
What do you notice?
What do you wonder?
What questions might a mathematician ask?
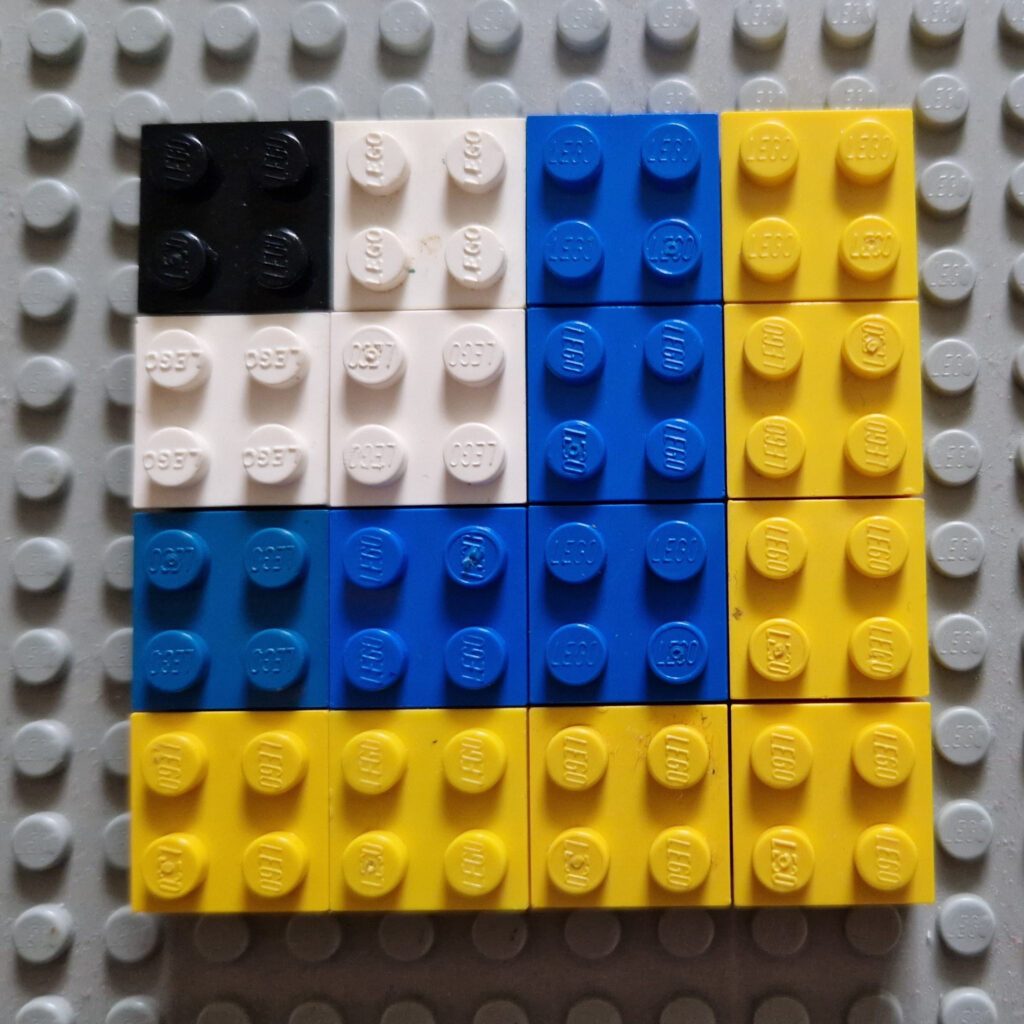
Can you draw or make the next pattern in the sequence?
How many bricks are there of each colour? How many bricks will you need of the next colour?