In the picture below, three congruent rectangles have been made in different ways with red and blue bricks.
The blue bricks are all arranged pointing up and down. The red bricks are arranged going across.
If we stick to this rule where blue bricks point in one direction and reds in the other, how else could you make this rectangle?
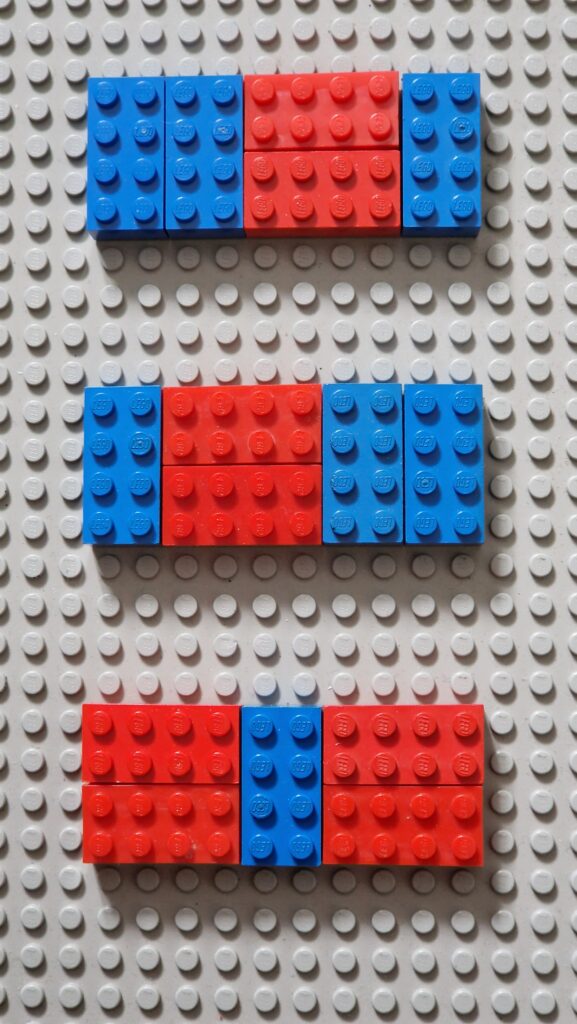
You might like to explore longer rectangles – this is the same size rectangle as five blue bricks, but we could make rectangles from six, seven or even more blue bricks. The bigger the rectangle you start with, the more ways there are of making it from a mixture of reds and blues in this way. Is there a pattern?
